Mathematics
When unfolded an origami model and marveled at the intricate crease pattern which forms the blueprint of the fold. Clearly there are some rules at play in these collection of creases. There is an origami geometry at work when paper is folded.
Such geometry, this mathematics of origami, has been studied extensively by origamists, mathematicians, scientists and artists. The Italian-Japanese mathematician Humiaki Huzita has formulated a list of axioms to define origami geometrically. Physicist Jun Maekawa has discovered some fundamental theorems about origami and used them to design origami models of surprising elegance. Mathematician Toshikazu Kawasaki has a number of origami theorems to his name and has even generalized some of them to describe paper folding in higher dimensions. (Origami in the fourth dimension) Robert Lang of California has developed an ingenious way to algorithmatize the origami design process, using a computer to help him invent models of amazing complexity. Educator Shuzo Fujimoto and artist Chris Palmer have discovered amazing parallels between origami and tessellations. And an enormous number of teachers have been developing ways to use origami to teach concepts in math, chemistry, physics and architecture.

Picture From : http://www.origami
-cdo.it/articoli/img/huzita.jpg
|
Humiaki Huzita : “Huzita-Hatori axioms”
What he described was called “Huzita-Hatori axioms” or “Huzita–Justin axioms”. It is the rule for folding paper. Huzita explain six distinctly different ways one could create a single crease by aligning one or more combinations of points and lines on a sheet of paper. Axiom 7 was discovered later by Koshiro Hatori, Jacques Justin and Robert J.Lang. |

Picture From : http://www.
papercrane.org/attendees/
JunMaekawa/portrait.jpg |
Jun Maekawa : “Maekawa Theorem”
Jun Maekawais credited with developing a new origami method based on fundamental geometric patterns. His insights into the connection between mathematics and origami are summed up in the Maekawa Theorem.
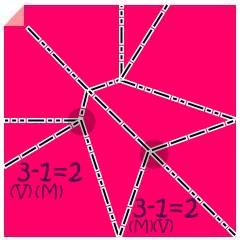
Maekawa's Theorem explains that the difference between the number of mountain folds(M)and the number of valley folds(V) in a flat vertex fold is always 2.
M – V = 2 or V – M = 2
For example
|
Toshikazu Kawasaki : Kawasaki
Toshikazu Kawasaki is an origami mathematician. He is known for the folding theory of planes and higher-dimensional folding styles as well as for creating the Kawasaki Rose series. He teaches mathematics at Sasebo College of Technology.
Kawasaki's theorem states that a given crease pattern can be folded to a flat origami if all the sequences of angles 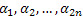 surrounding each vertex fulfils the following condition surrounding each vertex fulfils the following condition
Notice that the number of angles is always even and each of them corresponds to a layer of the folded sheet.
It basically means that the sum of every other angle about the vertex in a flat vertex fold is always 180 degree.

Picture From : http://www.curatedo
bject.us/photos/uncate
gorized/2008/01/03/
download2_5.jpeg |
Robert J. Lang
Robert J. Lang is known as one of the leading theorists of the mathematics of origami. He has developed ways to algorithmatize the design process for origami and discovered Axiom 7 after 6 axioms by Humiaki Huzita
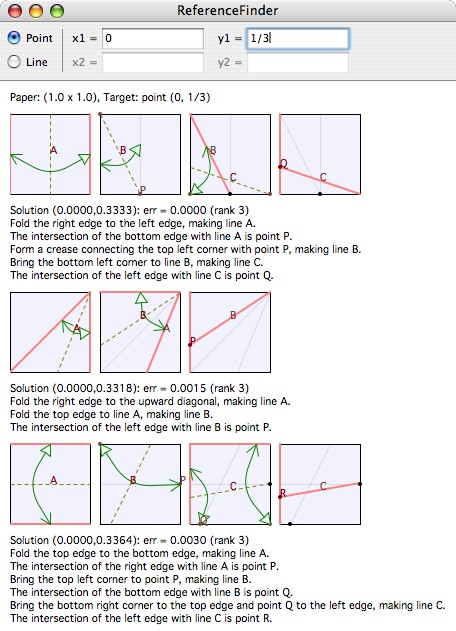
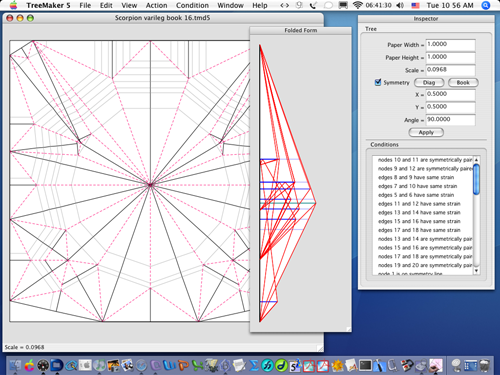
He use computer to solve origami design problems. The examples below are programs using for Origami folding developed by him.
|
- ReferenceFinder
- TreemakerTreemaker
- Origami Simulation
Source : http://kahuna.merrimack.edu/~thull/origamimath.html
http://www.langorigami.com
http://kahuna.merrimack.edu/~thull/combgeom/flatfold/flat.html
http://mathworld.wolfram.com/KawasakisTheorem.html
http://update.se-ed.com/265/origami-barking-dog-265.pdf
|